>> I HIGHLY SUGGEST GRABBING A
BOARD TO KEEP TRACK, THAT IS
BECAUSE THE MURPH CONSISTS OF A
MILE RUN FOLLOWED BY
100-PULLUPS, 200 PUSH UPS, 300
BODY WEIGHT SQUATS AND ANOTHER
MILE RUN A GRUELING EXPERIENCE
DURING IT YOU JUST KEPT
THINKING, JUST KEEP GOING KEEP
GOIONG THE RUN WAS REALLY
PRESSING AT THE END, WANTING TO
STOP AND WALK BUT I KEPT GOING,
MITCHELL AND MANY OTHERS KEPT
GOING TODAY
BECAUSE THEY SAY THEY HAVE A
VERY GOOD REASON TO PUSH
THEMSELVES TO THE LIMIT
TO HONOR MURPH
LT. MICHAEL MURPH MURPHY WAS A
NAVY SEAL, WHO LOST HIS LIFE IN
A FIREFIGHT IN AFGHANISTAN JUNE
28 2005 HIS LAST MOMENTS
DEPICTED IN THE MOVIE LONE
SURVIVOR.HE WAS PORTRAYED IN THE
FILM BY TAYLOR KITSC
>> IT'S THE LEAST WE CAN DO ONE
DAY A YEAR.
>> SOME COMPLETED THE WORKOUT
WITH 20 EXTRA POUNDS THE SAME
WEIGHT AS BODY ARMOR THE SAME
WAY LT.
MURPHY WOULD TACKLE IT.
>> WITH A WEIGHTED VEST I'LL SAY
IT SLOWED YOU DOWN QUITE A BIT
WHERE I HAD A PLAN GOING IN AND
THE PLAN CHANGED TO JUST KEEP
MOVING
>> WHAT IT BOILS DOWN TO IS THE
'MURPH' IS MORE THAN JUST A
WORKOUT.
IT IS NOW A TRADITION TO PUSH
US, HUMBLE, AND DEDICATE A BIT
OF PAIN AND SWEAT TO HONOR A MAN
WHO GAVE EVERYTHING.
THOSE WHO STEP UP TO THE
CHALLENGE DO NOT HAVE ANY
REGRETS.
>> ITS ONE OF THOSE THINGS THAT
SO MANY PEOPLE GET TO BE A PART
OF, IT DOESN'T MATTER IF YOU ARE
DOING HALF OF IT DOING WHAT YOU
CAN TO KIND OF SHOW YOUR
For more infomation >> Remembering Murph: Annual 'Murph' Challenge honors fallen Navy SEAL - Duration: 1:30.-------------------------------------------
Opel Corsa 1.3 CDTI ECOFLEX S/S COSMO - Duration: 0:58.
For more infomation >> Opel Corsa 1.3 CDTI ECOFLEX S/S COSMO - Duration: 0:58. -------------------------------------------
Toyota Auris 1.8 Full Hybrid Aspiration - Duration: 1:01.
For more infomation >> Toyota Auris 1.8 Full Hybrid Aspiration - Duration: 1:01. -------------------------------------------
Mazatlan Cattle Fair and its Dancers Horses - Duration: 12:31.
Horses and More
Visiting the Traditional Cattle Fair 2017, in Mazatlan Sinaloa Mexico.
You will be able to meet the farmers of Mazatlan who expose the best of their livestock.
Every year the farmers in Mazatlan enjoy this traditional fair.
Hi, how are you, my name is Anaha Esparza, I invite you to attend this fair in Mazatlan,
number 23, I am proudly representing Quelite, I invite you to come and vote for me in the red urn, thank you
Come and choose your favorite queen's candidate
Have a nice time at the village theater
Come and enjoy the fair in a family atmosphere
Hi, how are you, my name is Carolina Paez and I am a candidate for queen of this cattle fair,
I invite you all to come to know Mazatlan and our fair, my urn color is white, I invite you to vote for me, many Thank you.
Good evening, I invite you to come and meet the cattle fair of Mazatlan 2017,
come and vote in the pink urn, I represent Siqueros
Hello good evening, I am Lilia the outgoing queen I invite you to come and vote for the best, thank you very much.
You can not miss the dancers
We invite all people to come to Mazatlan Sinaloa to know our fair and the dancers we have, we have different
different breeds of horses, such as Frisians, Spanish, Iberoamerican, warlander, among others; Come and
meet us bring family and enjoy as we do, I am from here in Mazatlan Sinaloa, I am Dr. Ruben Osuna Lizarraga,
and I make a careful invitation to everyone to take us into account each May and come to know us And have fun with what we like.
I admire many beautiful horses
I hope you like them too
(♪♪♪ Song for a horse "The Marques" ♪♪♪)
♪♪♪ He was white-legged and had white forehead ♪♪♪
♪♪♪ Of 1.80 of elevation and a very elegant walk, for that reason horse friend, I will never be able to forget you ♪♪♪
♪♪♪ In the ranch of The Cardon of the port of Mazatlan ♪♪♪
♪♪♪ there is Magaña the owner of that Marques, the draft is not forgotten when he walked in the ♪♪♪
♪♪♪ goodbye cattle fairs, goodbye to regional festivities, goodbye to horseback riding, goodbye to carnivals,
Goodbye beautiful girls and I can never take them for a walk ♪♪♪
No doubt this is a good fair that leaves me wanting to return, and I recommend everyone to visit.
Month of May in Mazatlán Sinaloa, they are all invited.
Comment that you like this video, would you like me to make more videos like this?
Leave a Like if you liked it, share it and subscribe to the channel. It's free
-------------------------------------------
Geometry: Measurement of Angles (Level 9 of 9) | Examples VI - Duration: 5:08.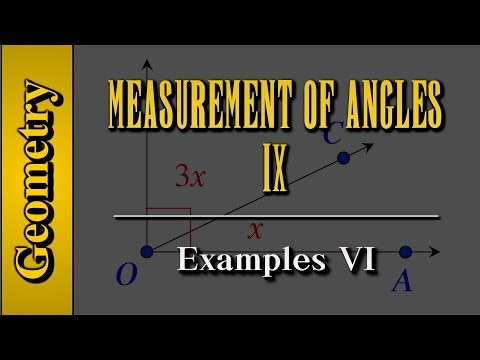
Measurement of Angles Level 9 In this final video on measurement of angles
we will go over 2 final examples that require the use of algebra to solve.
Let's take a look at the first example.
If angle POA is a right angle and if the measurement of angle POC is three times as large as the
measurement of angle COA.
Find the measure of angle POC.
In this problem we are provided with a figure along with geometric and algebraic relations
of two angles.
We are asked to find the measure of a particular angle.
We know from the problem that the measurement of angle POC is three times as large as the
measurement of angle COA.
So if we use the variable x to represent the measure of angle COA then the measure of angle
POC can be expressed as 3x.
From the diagram we know that the measure of angle POC plus the measure of angle COA
is equal to the measure of angle POA.
Using this geometric relation we can go ahead and substitute the algebraic and numeric expression
for the measurement of the angles.
We also know that angle POA is a right angle so we can replace the measure of this angle
with 90 degrees.
Now it is just a matter of solving for x, doing that we obtain x equals 22.5.
We can now use this value to determine the measurement of angle POC, substituting this
value into the algebraic expression and simplifying we obtain 67.5 degrees for the measurement
of angle POC and this is our final answer.
All right let's move along to the next example.
Given that angle TRS is a straight angle, angle TRX is a right angle, the measure of
angle TRS is 2x plus 5y, and the measure of angle XRS is 3x plus 3y solve for x and y.
In this problem we are provided with a figure along with 2 algebraic expressions one for
each angle.
We are asked to determine the values of x and y.
Notice that in this problem we actually want to solve for x and y just like we did in our
introductory algebra class.
The only difference is that we need to use the geometric relations to set up an equation
and solve for the variables.
Let's first label the diagram with the given information, we know that angle TRS is a straight
angle and measures 2x plus 5y.
We also know that angle TRX is a right angle.
So we go ahead and draw a square corner for this angle.
Since angle TRS is a straight angle the sum of the measure of angle TRX and XRS must equal
180 degrees.
Since the measure of angle TRX is 90 degrees we can conclude that the measure of angle
XRS is also 90 degrees.
Hence both angles are congruent meaning that both have the same measurement so the measurement
of angle XRS is 90 degrees.
Now we can equate the algebraic expression for the measurement of angle XRS with 90 degrees.
Notice that we now have 2 equations and 2 unknowns, at this point the problem becomes
a system of equations problem similar to the once covered in a typical beginning algebra
course.
Again if you are bit rusty on this topic I recommend you go back and review this topic.
We are going to use the elimination method to solve this system.
In this case we will eliminate the variable x.
So we need to multiply the first equation by 3 and multiply the second equation by negative
2, doing that we obtain the following.
Next we go ahead and add the equations.
Now that we have an equation with a single variable we go ahead and solve for y, simplifying
the expression we obtain 40.
The last step is to substitute this value into one of the original equations and solve
for the variable x.
Let's substitute this value into the second equation, solving for x and simplifying we
obtain negative 10 for the value of x.
So x equals negative 10 and y equals 40 so this is our final answer.
All right and we are now officially done with the measurement of angles video series.
In our next series of videos we will go over the concept of collinearity.
-------------------------------------------
wonder woman hair braid hair tutorial,braids hairstyle,haar vlecht,Tips by amal hermuz hair tv spain - Duration: 3:59.
For more infomation >> wonder woman hair braid hair tutorial,braids hairstyle,haar vlecht,Tips by amal hermuz hair tv spain - Duration: 3:59. -------------------------------------------
＜元AKB板野友美＞また整形疑惑！イメチェンをインスタでお披露目！画像あり - Duration: 2:31.
For more infomation >> ＜元AKB板野友美＞また整形疑惑！イメチェンをインスタでお披露目！画像あり - Duration: 2:31. -------------------------------------------
Rose se rend à la librairie Violette and Co - PAROLES D'ENGAGÉS 05 - Duration: 3:38.
For more infomation >> Rose se rend à la librairie Violette and Co - PAROLES D'ENGAGÉS 05 - Duration: 3:38. -------------------------------------------
Here's my Canada: The Four Seasons - Duration: 0:13.
I like Canada because I get to play lots of sports
and games. Also, because there are lots of famous attractions.
We have four seasons, winter, spring, summer, and fall.
-------------------------------------------
Here's My Canada: Many Attractions - Duration: 0:08.
What I like about Canada
is that there are a lot of places to go.
-------------------------------------------
Aurora Sewer Line Clogs | 815-630-1024 | Aurora IL Sewer Line Clog - Duration: 1:04.
Aurora Sewer Line Clogs. Are you sick of having your drains or sewer line clogged and having to pay a plumber every
6 months to come clear it out?
Hydro jetting is a long lasting solution to the problem of drain obstructions and tree
roots intruding into sewer lines.
We have a state of the art high pressure water jetter that cleans out grease, sludge, tree
roots or any other blockages in your pipes.
While conventional snaking only pokes a hole in the clog, water jetting cleans out the
entire surface of the pipe.
We are trained experts in sewers, drains, and septic systems.
We'll stop your problem at it's source and keep your home safe.
To get a better view of what's going on, our technicians can do an in-pipe camera inspection.
If your drain is blocked and causing issues, emergency service is available.
Give us a call today, we'll get there fast!
No comments:
Post a Comment