MorphineBear
Thanks for subbin'.
Is this dumb anime music?
It's not anime music, I don't think.
Why you gotta call me "pus C?"
Ooohhhh yeaaaaaaah.
This guy on Twitter was like:
"I'm thinkin' about subbing Moonmoon..."
I was like, "do it pus C."
For more infomation >> PARHAAT PALAT (2016) w/English subtitles - Duration: 9:59. 
For more infomation >> Check Out We Love You
For more infomation >> Madczech Apokalipse Epizode 01 - Duration: 1:33. 
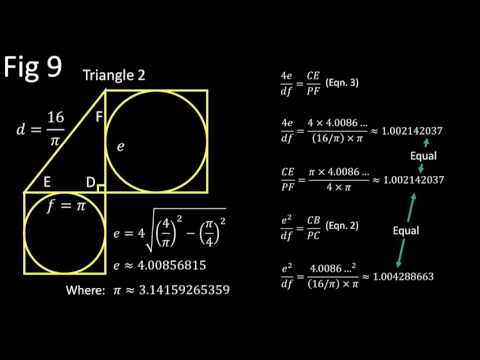
Okay, I realize that at the end of my critique on "The Great Pi Conspiracy,"[1] I challenged
my viewers to look over Mark Wollum's two articles and "find the ever-so-slight flaws
for [yourselves]." Problem is, some people are not able to think
for themselves and need to be led around by the ring in their nose.
That's one reason why I'm against body piercings. YouTube user, C No-one, for example, had a
hard time following along while I explained that you can't just claim two right triangles
are similar. You have to prove they're similar in some
way BEFORE you start using properties that are only true for similar triangles.
Similarly, you have to prove that two triangles are Kepler triangles BEFORE using properties
that are true only for Kepler triangles. And, you can't prove you have a Kepler triangle
by using a relationship that is true for more triangles than just Kepler triangles.
And you certainly can't prove a triangle is a Kepler triangle, or that you have two similar
triangles, if you have to change a constant value to do it.
I even demonstrated, using triangles that are clearly not Kepler triangles, that you
can force any triangle to pass Wollum's "side length test" by changing known constants.
But somehow, C No-one, didn't get it.
So, I'm guessing that he won't get this video either.
In "The Great Pi Conspiracy, Part 2,"[2] Wollum starts with two new triangles, both with a
hypotenuse of 16 over pi. Redrawing his triangles in a more standard
form, you can make a good guess at what Wollum's intent will be.
He will probably want to make these two triangles congruent, meaning equal sides and angles.
Of course, using what little information is available (just two different sides for each
right triangle), you can easily show that the corresponding angles of these two triangles
are not equal; therefore they cannot be congruent, or even similar.
Oops! I think we've lost poor C No-one already. Also, using the known value of pi, we can
calculate the missing sides and show that they are not the same, further proving that
the two triangles are not congruent. But, the short side of triangle 1 is CLOSE
to pi and the long side of triangle 2 is CLOSE to 4, so they look nearly the same.
Then, if you ASSUME that the ratios of the known sides are equal to the ratios of a Kepler
triangle, you come up with the relationship that Wollum wants us to believe.
Problem is, Wollum never establishes that he has two congruent Kepler triangles BEFORE
arriving at his new value of pi. And, the only way he could do that is by calculating
the ratios, which only proves neither triangle is a Kepler triangle.
Now, folks who suffer from conspiracy mentality don't understand the necessity of starting
with known facts before venturing into the unknown.
They make up their own story as the go along and often use logic that's not very logical.
C No-one, for example, thinks it's a fallacy to use the traditional value of pi in the
calculations I've just shown, but it's NOT a fallacy to use Wollum's proposed pi.
Of course, you can't have it both ways. If you can't use one value, you can't use
the other. You need to find another approach to demonstrate
that the two triangles are similar, or congruent, or Keplerian, that doesn't use the value pi
to do so. Wollum failed to do this beforehand.
I'm going to skip Wollum's figures 2 through
6 that are just background that we covered in part 1, and jump right in to where Wollum
begins his "proof" for his new value of pi. Now, if this first equation makes about as
much sense as: 5, times 8 over 5, times 3 over 8, equals 3, don't worry.
There's nothing wrong with you. It didn't make much sense to me either.
All Wollum is doing is finding a path to what he calls equation 1, a over c equals the perimeter
of square B over the circumference of circle C.
Now, I would have taken a more direct approach using the a over b identity Wollum gives us
in his figure 7. But, in this situation, I suppose it's not
the journey that's important, just the destination.
Here Wollum does some real hocus pocus. In step 2, he multiplies each side by pi squared
over 16. That's okay.
We can multiply each side of an equation by the same constant or variable for that matter
and it doesn't change the equation. This constant simply gets sucked into the
variable ratio on the right, but in step 5, he commits an inappropriate substitution that
would make Mr. Spruell cringe. Wollum notes that we know b over a equals
pi over 4, right? Then, it follows that b squared over a squared
equals pi squared over 16, right? So, anywhere we see pi squared over 16, we
should be able to substitute b squared over a squared, right?
Wrong. You can't just go around willy-nilly, changing
constants into variables or ratios of variables for that matter, especially, if you don't
know where those constants have been. You might as well pick up candy off the sidewalk
and eat it. Constants are fixed values.
You can't change constants. They are specific.
Variables represent values that CAN change. They are not specific.
When you know what a variable's value is, you can replace that variable with its value,
but not the other way around. Not without suffering consequences.
In this case, Wollum essentially multiplied one side of the equation by a variable expression
and the other by its value in step 2. Not apropos, but the only way to force equation
2 out of this mess. Actually, I couldn't figure out anyway to
get to equation 2 without replacing 4 with b somewhere.
So, Wollum's statement that equation 2 is "Valid for a set of triangles which all have
the same interior angles," is incorrect. The equation is only valid when b equals 4,
because that's the condition he locked us into when he made the inappropriate substitution.
But, of course, Wollum knew that. Now, take a close look at equation 2.
Déjà vu all over again, right? Where have we seen something like this before?
Well, back in part 1 and repeated in figure 6 in part 2, Wollum gives us the "special
property" for Kepler triangles, a times c equals b squared.
Well, that would make b squared over a c equal to 1, wouldn't it?
It would then directly follow that the length of side c is equal to pi.
This would be true IF triangle 1 was a Kepler triangle, which Wollum never proves beforehand.
Wollum takes another circuitous route around triangle 1 to get to his equation 3.
In the process, he makes a second inappropriate substitution, replacing b squared with 4 times
b in step 2. Oh, the humanity!
Surprisingly, you can get directly to equation 3 from a known identity, a (the hypotenuse)
equals 16 over pi, without any inappropriate substitutions whatsoever.
So, equation 3 is actually a valid equation, not only for triangle 1, but for ANY triangle
that has a hypotenuse equal to 16 over pi. Yes, totally VALID!
There's absolutely nothing wrong with equation 3.
Unfortunately for the pi conspirators, equation 3 is a balanced equation (meaning both sides
evaluate to the same value) for the real value of pi, 3.14159-whatever.
And, coincidentally, because b equals 4 in triangle 1, equation 2 is also necessarily
a balanced equation using the real value of pi.
How about that? Actually, truth be known, both equations are
balanced for pi equal to ANY positive number less than 4, which includes the real value
of pi and also Wollum's new pi. I'll leave it as an exercise for the casual
student to show why that is. But, since equation 3 is valid for ANY triangle
with a hypotenuse of 16 over pi, then it's balanced over the same range of pi when applied
to triangle 2. How convenient, huh?
Of course, for triangle 2, equation 2 will only have one solution for pi, which will
result in the required prerequisite, b = 4. Can you guess what that value might be?
Nevertheless, you can see where Wollum could apply his equation 3 to triangle 2 and get,
not surprisingly, b equals 4, regardless of what value he assigns to pi, because pi literally
drops out of the equation. But, of course, Wollum knew that.
Now, for the life of me, I couldn't understand
the point behind Wollum's sidebar on similar triangles.
He might just as well have gone, "Look, squirrel," and then continued on with his analysis.
Without getting sucked into the details, his conclusion is that equation 2 can only be
valid for right triangles with "specific internal angles," where the ratio of the hypotenuse
over the long side equals 4 over pi. And, the same goes for equation 3.
Of course, the angles DO change if you change the value of pi.
With respect to triangle 1, if you let the value of pi increase, the length of the hypotenuse
and the short side both decrease in length and the smaller acute angle also decreases.
Likewise, for triangle 2, as pi increases, the hypotenuse and the long side both decrease
in length, while the short side and the smaller acute angle both increase.
The angles change because the lengths of the sides change when you change the value of
pi. Bottom-line, equations 2 and 3 are linked
to the hypotenuse of the triangle and have nothing to do with any congruent angles.
But, of course, Wollum knew that.
Wollum then performs another bizarre conversion to show you can derive his equation 3 directly
from triangle 2. Of course, his approach requires yet another
inappropriate substitution, swapping f for pi in step 5, as if that would surprise anyone
at this point. But, we already know that equation 3 is valid
for ANY triangle with a hypotenuse equal to 16 over pi.
Triangle 2 is simply one of many. Yet, somehow, Wollum concludes that since
he could derive equation 3 from both triangles, then (Look, squirrel!) both triangles must
have the same interior angles and therefore they must be congruent.
Sur-prise, sur-prise, sur-prise!
Then, for his coup de grâce, Wollum shows that, for his hybrid triangle, the ratio of
the hypotenuse over the long side equals the ratio of the long side over the short side,
and therefore (Look, squirrel!) this must be a Kepler triangle and therefore pi must
be equal to 4 over the square root of the golden ratio.
Betcha' didn't see that comin'. Of course, equal ratios, like Wollum's "special
property," don't necessarily mean you have a Kepler triangle.
In fact, it doesn't even mean you have a right triangle at all.
And simply finding a solution to a quartic equation, which (déjà vu) we solved in
part 1 doesn't mean the solution is some profound revelation of an ancient conspiracy to hide
the true value of pi from the general public. It just means that people who have a natural
tendency toward confirmation bias will ignore inconvenient facts.
They see "evidence" where there is none and think that it supports their preconceived
conclusions, even if the "evidence" contradicts their beliefs.
Often, they project themselves on others, pushing their own cognitive biases onto other
people.[3] They miss the relationship between words like
"coincide" and "coincidence," and make up new meanings to words like "derive" and "derivation"
to fit their argument. And no matter how good a job you do to prove
them wrong, no matter how clear and rational your argument, they never seem to understand.
But, it's not their fault. They have a mental block, some kind of defense
mechanism that protects their own confidence in their fragile convictions.
At this point, I don't know who's more insane, the people who clearly suffer from psychological
disorders that make them vulnerable to believing junk science and conspiracies, or the people
who argue with them. Tough call, that one.
Subscribe to:
Post Comments (Atom)
No comments:
Post a Comment